本文是数学专业的留学生作业范例,题目是“How Does the Idea of Limits Make the Calculus Precise?(极限的概念如何使微积分精确?)”在这篇文章中,我将讨论微分的主题,以及微积分的思想是如何受到批评的。我将概述如何解释微积分以及如何解释极限和无穷小数的概念。牛顿和莱布尼茨之间的微积分争议是已知的最伟大的数学争议之一,因为这些发现的重要性和成就。相反,他们的发现引入了微积分的基础,也就是通常所说的微分和积分。他们的发现受到了很多人的严厉批评,尤其是数学家伯克利。尽管伯克利承认了微积分的真理,但他很快就反驳了他们的理论。伯克利本人就是一位伟大的数学家,后来他的工作就是找出别人工作中的缺点和裂缝,并公开揭露它们。伯克利接受了微积分的思想,但由于无穷小的使用,他发现这个思想存在问题。
In this essay, I will be discussing the topic of differentiation and how the ideas of the calculus were criticised. I will be giving an overview of how calculus can be explained and explaining the idea of limits and the infinitesimal number.
The Calculus controversy between Newton and Leibniz is known to be one of the greatest mathematical disputes due to the importance and accomplishment of such findings. On contrary, their findings introduced the foundations of calculus which is more commonly referred to as differentiation and integration. Their findings were criticised heavily by many people but especially a mathematician known as Berkeley. Yet Berkeley acknowledged the truths derived from calculus, he was quick to pounce and to try disproving their theories. Berkeley was a great mathematician himself, subsequently his work was to find faults and cracks in other people’s work and publicly expose them. Berkeley accepted the ideas of calculus but found the idea problematic due to the use of the infinitesimal number.
(Wisdom ,1953. pp. 22-25) denotes that philosophers supposed that the purpose of Berkley’s findings was to indulge in a futile trial of strength with newton, this can suggest that Berkeley was jealous of newton but can also suggest that he cared more about taking newton down rather than the mathematics involved. Wisdom also pointed out that Berkeley attacked the logic of the infinitesimal number saying that the value of zero is seen as a finite quantity of no size and also a zero-increment value. Due to the quantity of zero not being consistent, Berkeley dismissed this problem as “ghosts of departed quantities”. This idea means that the effects of zero being infinitesimally small and zero itself suggests that newtons work was self-contradictory and the effects of this remained in the calculations after it was supposedly made to vanish. However, Berkeley didn’t understand that newtons findings were only the starting foundations with an expansion to the theory of limits. Wisdom goes on to explain that despite the destructive criticism of Berkeley, it was extremely important as the errors he investigated were compensated for with the introduction of limits and the movement away from the infinitesimals.
(Grabiner 1997. pp. 393-394) insinuates that Berkeley attacked the logical validity of the calculus adding to his assault on Newton as a person and a mathematician. Berkeley is described to be witty and unkind when criticising newtons mathematical practises. Berkeley also accuses mathematicians of being hostile to religion which is considered to be irrelevant when confronting a mathematical theory. Subsequently, Berkeley’s approach to his challenge on newton was not a mathematical one, yet an attack on him and the people who supported newton.
Theoretically speaking in terms of real numbers, the infinitesimal number is missing as it is not regarded as a real number. Infinitesimal numbers can be referred to as quantities that approach to zero or can be expressed as variable quantities. The word infinitesimal means that something is so small that it is impossible to measure or calculate. An infinitesimal object is one that cannot be measured feasibly but does never equal zero.
从理论上讲,从实数的角度来说,无限小的数字是缺失的,因为它不被视为实数。无穷小数可以被称为接近于零的量,也可以被表示为变量。无穷小这个词的意思是某物太小以至于不可能测量或计算。一个无穷小的物体是指一个不能被可行地测量但却不等于零的物体。
Limits are a measurement that stays in our dimension and maintains the supposedly perfect illusion of such model. Infinitesimals are much smaller and accurate than limits in such that models are built in another dimension, much more accurate and smaller and maintains the illusion perfectly in the normal dimension.
Infinitesimals have a rigorous foundation.
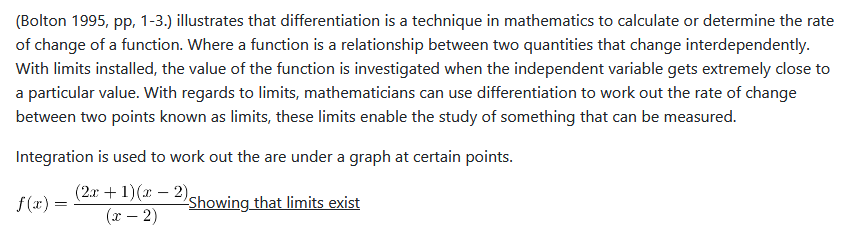
If we take the function,
For when x=2.
If we sub in 2 for x, then the function gives an infinite number, this Is because the numerator which equals 5 is divided by 0 which cannot be calculated as there are infinite numbers the answer can be.
Alternatively cancelling out the (x-2) yields the same denominator of 0. For this to happen x cannot be equal to 2. it must be equal to anything other than 2 to cancel out the same terms of (x-2). Then we are left with:
如果我们用2代入x,那么函数会得到一个无限数,这是因为分子等于5除以0这是无法计算的,因为答案可能是无限数。
或者消去(x-2)得到相同的分母0.此时x不能等于2.它必须等于除2之外的任何数才能消去(x-2)的相同项。然后我们得到:
f(x)=(2x+1)
This simpler function can now predict that;
f(2)=5
So, the prediction for this even is 5. this number was calculated using behaviour outside of the event to predict the actual outcome behaviour of the event.
To work out the validity of the event, we need to input an accuracy threshold or a range to stay within the given accuracy.
For example, I will place an accuracy threshold of ±1 so will give answers either side of 5. so will be 4 and 6. For this to be, x must equal 1.5 or 2.5 to produce such numbers. As a result, this function is said to limit 2. this is because when x equals 2. the function depicts an infinite nature. Thus, f(x) can be made extremely close to the limit of 2 just by making x especially close to 5.
例如,我将设置一个准确性阈值±1.所以将给出答案的任何一边为5.也就是4和6.对于这个,x必须等于1.5或2.5才能产生这些数字。因此,这个函数的极限是2.这是因为当x = 2时。这个函数描述了一个无限的性质。因此,只要让x特别接近5.f(x)就可以非常接近2的极限。
In chapter 4 of ‘MATHEMATICS A VERY SHORT INTRODUCTION’ , Gowers talks about the ideas of limits and infinity and explains to how to deal with such difficulties. He starts with talking about the concept of the square root of a number. Gowers illustrates that the square root of 2 is roughly 1.41421356 and explains that this number is accepted to being equal. The root of 2 lies between 1.4 and 1.5 and so the decimal expansion starts.
1.41421356²=1.9999999933878736
1.41421357²=2.0000000216721449
If the decimal expansion keeps increasing there will be an infinite increase of the number 9 so 1.9999999… recurring is considered to be equal to 2; this is an accepted truth of mathematics.
Another scenario Gowers investigated was one associated with speed, distance and time. Gowers illustrated to his audience a scenario that shows calculus cannot calculate the average of speed of a car but can calculate the average speed of a car during that time over a distance. Consequently, this does not tell you the speed of a car at any moment in time. In order to overcome this hurdle, Gowers talks using infinitely small periods of time and calculating how many miles the car has travelled in such small time. This “ridiculous fantasy” as Gowers defines it creates numerous problems in the mathematical world and portrays similar problems to such ideas that 1.9 recurring is the same as 2. Subsequently, working out the speed of a car in such a small time is regarded as invalid when a time of 0 seconds is used. However, exploiting the fact that in a small period of time, cars do not actually accelerate much at all, so using accurate measuring devices and an appropriate time frame, a close approximation can be calculated and yet wont yield the correct answer, but will provide us with an answer mathematically correct. This is also reminiscent of mathematical models in the fact that they yield a scientifically accepted approximation of a natural phenomenon but is also not utterly one hundred per cent accurate.
Infinity is involved once again and due to its infinite nature, limits are suggested to be put in place which is a sequence of finite decimals that can be used to calculate the approximation for the scenario that involves an infinite decimal count.
Gowers then goes onto explain the calculations that involve the area of the circle using ϖr². The area of an object is described as the “amount of stuff” that a shape beholds. The issue regarding the calculations of the area of circles is due to its curved nature, therefore, it is impossible to fit triangles into such shapes. A circle never has a straight line and as triangles do, there will always be a small area unaccounted for as shown in the diagram to the left, unless an infinite number of thin triangle is used, which is understandingly impossible to calculate.
然后Gowers用ϖr²解释了涉及圆面积的计算。物体的面积被描述为一个形状所看到的“物质的数量”。关于圆面积的计算问题是由于其弯曲的性质,因此,不可能将三角形放入这种形状中。圆永远不会有直线,就像三角形一样,总是会有一小块区域无法计算,如左图所示,除非有无数个细三角形被使用,这显然是不可能计算出来的。
Gowers estimates the area of this circle using numerous squares, the main problem is that many squares lie partly inside the shape. The obvious way to improve the accuracy of the estimate is to make the squares smaller, yet the result of this just makes the approximation closer to a specific number that cannot be worked out due to the infinite number of squares that there has to be to be distributed over the whole circle. The area of a circle is mathematically accepted if a tolerable percentage of error is used. To get the exact final answer, an infinite amount of infinitely small squares must be used which is impossible to calculate.
If you draw a straight tangent to the circle at any point on the circumference, the gradient of the tangent will equal the gradient of the circle line at an infinitely small value, therefore there would need to be an infinite number of squares for there to be an accurate calculation of the circle area.
如果你直接画一个圆的切线在圆周上任意一点,切的梯度等于圆的梯度线在一个无限小的价值,因此,需要无限的广场有一个准确的计算圆的面积。
To conclude on this overview of chapter 4. Gowers has provided us with a “fruitful” interpretation about the ideas of infinity and the use of limits and how they can provide us with an approximation rather than defining a scenario completely in one go. (Gowers, 2006. pp56-69.)
Differentiation is the process of acquiring the derivative of a function or working out the rate of change of an event; for example, the acceleration of a car or the rate of change with respect to x and y on a graph/ the gradient of the line.
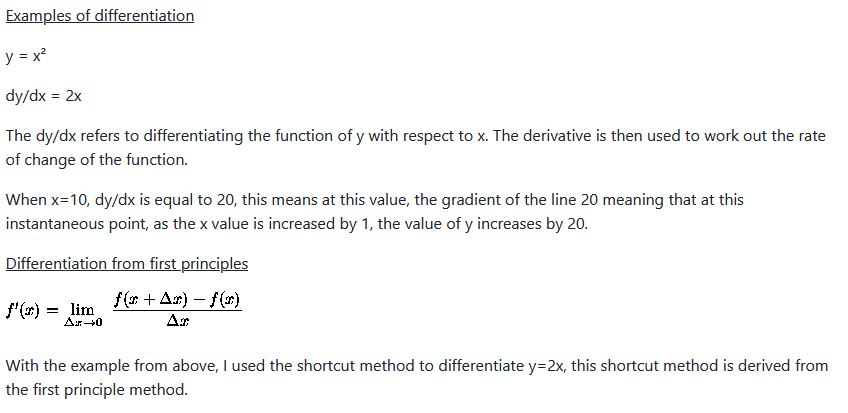
With the example from above, I used the shortcut method to differentiate y=2x, this shortcut method is derived from the first principle method.
For example:
For y=x²
f‘x=limfx+h–fx /h
as h limits to 0.
f‘x=lim(x+h2)–fx² /h
as h limits to 0.
f‘x=lim(x2+h2+2xh–x²) /h
as h limits to 0.
f‘x=lim(2x+h)
as h limits to 0
f‘x=2x
As h limits to 0. this means that the value of h can be infinitesimally small but will never equal to due to the fact that the equation cannot be divided by 0. ‘h’ can never be 0 because differentiation is the rate of change between two variables and if h equals to 0. it suggests there has been no change, so differentiation cannot take place.
当h趋于0时。这意味着h的值可以是无限小的,但永远不会等于,因为这个方程不能除以0.h永远不可能是0.因为微分是两个变量之间的变化率,如果h等于0.它表明没有变化,所以分化不会发生。
To conclude, Leibniz and newton introduced the foundations of calculus and although it was heavily criticized for its validity, it is widely used today and is being taught to millions of children in schools every year all over the World. Their findings are substantial in the mathematical world but if it wasn’t for the thorough assessment and criticism of their work by Berkeley, then calculus wouldn’t be fully intelligible to what it is today. Limits are a way of expressing a function that gets extremely close to a number until it has an infinite number of solutions. Newton and Leibniz graced us mathematicians with the brilliance of differentiation and integration and enabled other more recent mathematicians to venture on to different sub topics and develop their understanding of calculus.
总之,莱布尼茨和牛顿介绍了微积分的基础,尽管它的有效性受到了严厉的批评,但它今天被广泛使用,每年在世界各地的学校里被教授给数百万儿童。他们的发现在数学世界中是非常重要的,但如果不是伯克利对他们的工作进行了彻底的评估和批评,那么微积分就不会有今天的样子。极限是表示一个函数的一种方式,它非常接近一个数,直到它有无限个解。牛顿和莱布尼茨以微分和积分的卓越才智使我们的数学家们感到光荣,并使其他近代的数学家们能够冒险研究不同的子课题,并发展他们对微积分的理解。
数学作业相关专业范文素材资料,尽在本网,可以随时查阅参考。本站也提供多国留学生课程作业写作指导服务,如有需要可咨询本平台。
